Simply put, the sun puts out most of its energy in the visible spectrum. So the obvious explanation is that our eyes adapted to where the light is strongest. That would be the end of the story except for the fact that atmosphere is transparent to visible light. It has an open window at just the right place. One possibility is that the atmosphere “evolved” in the sense that plants that produced the right atmosphere to let the visible light in thrived and an evolutionary feedback loop optimized our atmosphere for the transmission of visible light. In any case it should be remembered that there are two happy circumstances to explain: the sensitivity of our eyes to the peak of the sun’s distribution and the hole in the atmosphere at just the right place.
I don’t know it, but it us a universally accepted assumption from every scientists I know, regardless of their theism or atheism. If you think some kind of life is possible from the products of the big bang, you are in a fringe minority. That’s really science fiction, not science. And there are many places in the universe that you could look for such life–vast hydrogen clouds. I don’t think you could convince anyone searching for extraterrestrial life to look there as opposed to near stars where where are lots of heavy elements.
If life, any kind of life, requires the storage of information, then you won’t find it without heavy elements.
Absolutely not. The region is considered small if small fractional changes in the constants result in a loss of habitability. That statement is completely independent of any assumed distribution. If (as a made-up example) a constant must be 1 plus or minus .01, as opposed to 1 plus or minus 100, then we would say that habitability appears to be sensitive to that value. It doesn’t matter scientifically (although it might philosophically) whether the value came from a random draw and was therefore highly improbable, or the number was dictated by a fundamental theory. Those are two extreme distributions, but in both cases we still have sensitivity and therefore “fine tuning.”
Of course the explanation for the sensitivity would depend on which case is correct. Or perhaps the other way around. The multiverse, for example, favors a large number random draw low-probability scenario. (Which is why it is so odd that IDers also want to argue low probability.)
I’m not sure there’s something there to be explained. Had the “hole” been elsewhere, eyes would have adapted to whatever radiation slipped through there instead. You might say there’s much less radiation coming through elsewhere, but it seems to me that’s only a problem if it’s so little it makes sight useless. Afaicg eyes would work on Mars, and Venus, and basically any other body in the solar system with an atmosphere. Is there some imaginable planet with a combination of an atmospheric composition and a star’s spectrum that would make vision impossible, short of a layer of ash?
You are obviously entitled to that view. I’ll continue to be grateful that evolution did not have to choose between a transparency window and the peak of the sun’s spectrum.
Sadly, constants can be stated in different ways, with great variation in the magnitude of change. Consider, for example, replacement of a constant by its reciprocal.
I’ll take that as a no.
I am sorry, but that is simply not a legitimate argument.
I would think this obvious for no other reason than physicists of all stripes consider the appearance of fine tuning to be an interesting and serious puzzle that demands a scientific explanation. They are pretty smart when it comes to math. I don’t think they’d agree with the argument that if habitability appears to be sensitive to the value of a constant, we’ll simply state the constant in a different way.
This reminds me of an argument that I once heard: if the cosmological constant is inexplicably, unimaginably, and necessarily small (as it appears to be) then we can just choose different units, where it’s not so small.
Well, that’s just confused.
Do they? Or are they interested in things that happen to bear some surface similarity to fine tuning? Are fundamental constants connected to each other? Could the be different? What would be the effect if they were different? I don’t see any of these questions as involving a fine-tuning hypothesis.
What, in that sentence, does “small” mean and how can you determine that it’s small without reference to some standard of size?
Small means it (the cosmological constant) is roughly 100 orders of magnitude less than the best calculations suggest. Nobody has an explanation for the cancellation “conspiracy” required to achieve the result. And yet a small cosmological constant appears to be necessary for habitability, lest the universe expand too rapidly to form galaxies and stars.
If you subtract two large numbers and the difference is dozens of orders of magnitude smaller than the large numbers, but not zero. So the difference is small compared to the numbers. We don’t have a good explanation why, and it is very weird.
That’s why talk of changing units is so absurd. We are discussing a ratio here, which is unitless.
My experience of the term is quite different. That surely might mean that the people who use it (in my experience) were confused (or dishonest) about what fine tuning is about. But IMO fine tuning would not be valuable apologetically if it were only about “the constants of our universe” and not about their likelihood.
Well then, you have a standard. What are these calculations?
One could, however, invert the ratio, and then it becomes a large number. Why prefer one over the other?
Likelihood seems to have been introduced. Both @david.heddle and @swamidass are saying that such a small number is surprising ('nobody has an explanation" and “weird”), which is a very small step from unlikely.
I’m not making a fine-tuning argument, just trying to explain what some of the actual puzzle really are here… Perhaps reading up a bit about the hierarchy problem in physics might be helpful:
A simple example:
Suppose a physics model requires four parameters which allow it to produce a very high-quality working model, calculations, and predictions of some aspect of our physical universe. Suppose we find through experiments that the parameters have values:
- 1.2
- 1.31
- 0.9 and
- 404,331,557,902,116,024,553,602,703,216.58 (roughly 4×10^29).
We might wonder how such figures arise. But in particular we might be especially curious about a theory where three values are close to one, and the fourth is so different; in other words, the huge disproportion we seem to find between the first three parameters and the fourth. We might also wonder, if one force is so much weaker than the others that it needs a factor of 4×10^29 to allow it to be related to them in terms of effects, how did our universe come to be so exactly balanced when its forces emerged? In current particle physics the differences between some parameters are much larger than this, so the question is even more noteworthy.
I don’t think that. I simply don’t know. I’m rejecting your claim that it isn’t.
If.
Field theory calculations of the cosmological constant.
Surely you must see the ramification of this, were it true. It would mean that the more unhappy a scientist was because of the large uncertainty in their result, the easier they could solve the problem by reporting the reciprocal of their result.
As I said several times, it is surprising that the habitability is so sensitive to the value regardless of the probability of the value (which nobody can calculate anyway). It is the sensitivity that is surprising, not the probability of the value.
Lawrence Krauss also has a short explanation for the absurdity of that value in this video, beginning at about 19:00 minutes in:
The atmosphere has lots of open windows.
The sea doesn’t.
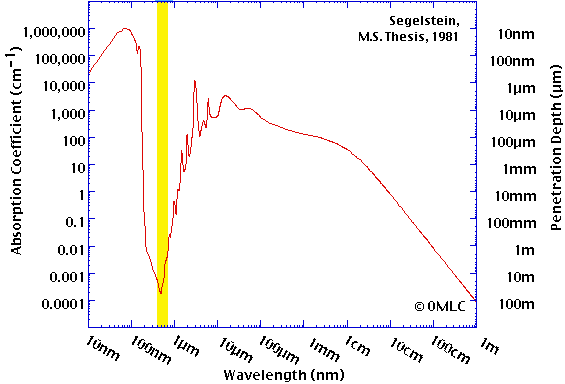
Eyes are sensitive to the spectrum where seawater most transmits light.
Of course, not all eyes have the same spectral range. Birds, insects and most fish can see into the ultraviolet range. This isn’t surprising since both the solar and marine spectra extend in that direction.
There’s just one - the match between the solar radiation and the marine transmittance spectrum. The sensitivity of eyes to that range is thus explained.
(I don’t think seawater “evolved” in the sense that marine life produced the right seawater to match the solar spectrum.)
He does not have an explanation for the absurdity of the value, he is (I’m guessing, because I only watched a little) explaining the interpretation of the cosmological constant as the energy of the vacuum and dark energy. If he had a testable explanation for the absurdity of the value (other than an anthropic explanation-- if weren’t so small we wouldn’t be here to talk about it) I believe he would win a Nobel Prize.
In fact, quite to the contrary, it is this same (anti-theist) Krauss who described the smallness of the Cosmological Constant as the “greatest fine tuning problem in all of physics” which you can easily Google-verify.
My only nit with that statement is that whether it is a problem or a feature is a matter of perspective.

